Abstract
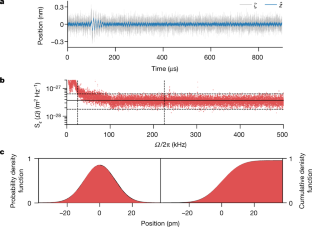
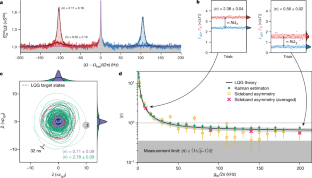
The ability to accurately control the dynamics of physical systems by measurement and feedback is a pillar of modern engineering1. Today, the increasing demand for applied quantum technologies requires adaptation of this level of control to individual quantum systems2,3. Achieving this in an optimal way is a challenging task that relies on both quantum-limited measurements and specifically tailored algorithms for state estimation and feedback4. Successful implementations thus far include experiments on the level of optical and atomic systems5,6,7. Here we demonstrate real-time optimal control of the quantum trajectory8 of an optically trapped nanoparticle. We combine confocal position sensing close to the Heisenberg limit with optimal state estimation via Kalman filtering to track the particle motion in phase space in real time with a position uncertainty of 1.3 times the zero-point fluctuation. Optimal feedback allows us to stabilize the quantum harmonic oscillator to a mean occupation of 0.56 ± 0.02 quanta, realizing quantum ground-state cooling from room temperature. Our work establishes quantum Kalman filtering as a method to achieve quantum control of mechanical motion, with potential implications for sensing on all scales. In combination with levitation, this paves the way to full-scale control over the wavepacket dynamics of solid-state macroscopic quantum objects in linear and nonlinear systems.
Access options
Subscribe to Journal
Get full journal access for 1 year
199,00 €
only 3,90 € per issue
Tax calculation will be finalised during checkout.
Rent or Buy article
Get time limited or full article access on ReadCube.
from$8.99
All prices are NET prices.

Data availability
The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.
References
- 1.
Åström, K. J. & Wittenmark, B. Computer-controlled Systems: Theory and Design (Courier Corporation, 2013).
- 2.
Geremia, J., Stockton, J. K., Doherty, A. C. & Mabuchi, H. Quantum Kalman filtering and the Heisenberg limit in atomic magnetometry. Phys. Rev. Lett. 91, 250801 (2003).
- 3.
Glaser, S. J. et al. Training Schrödinger’s cat: quantum optimal control. Eur. Phys. J. D 69, 279 (2015).
- 4.
Wieseman, H. M. & Milburn, G. J. Quantum Measurement and Control (Cambridge Univ. Press, 2010).
- 5.
Sayrin, C. et al. Real-time quantum feedback prepares and stabilizes photon number states. Nature 477, 73–77 (2011).
- 6.
Yonezawa, H. et al. Quantum-enhanced optical-phase tracking. Science 337, 1514–1517 (2012).
- 7.
Jiménez-Martínez, R. et al. Signal tracking beyond the time resolution of an atomic sensor by Kalman filtering. Phys. Rev. Lett. 120, 040503 (2018).
- 8.
Carmichael, H. An Open Systems Approach to Quantum Optics (Springer, 1993).
- 9.
Kalman, R. E. A new approach to linear filtering and prediction problems. J. Basic Eng. 82, 35–45 (1960).
- 10.
Kalman, R. E. et al. Contributions to the theory of optimal control. Bol. Soc. Mat. Mex. 5, 102–119 (1960).
- 11.
Sittig, D. F. & Cheung, K.-H. A parallel implementation of a multi-state Kalman filtering algorithm to detect ECG arrhythmias. Int. J. Clin. Monit. Com. 9, 13–22 (1992).
- 12.
Bar-Shalom, Y., Li, X. R. & Kirubarajan, T. Estimation with Applications to Tracking and Navigation: Theory Algorithms and Software (Wiley, 2004).
- 13.
Ruppert, M. G., Karvinen, K. S., Wiggins, S. L. & Moheimani, S. O. R. A Kalman filter for amplitude estimation in high-speed dynamic mode atomic force microscopy. IEEE Trans. Control Syst. Technol. 24, 276–284 (2016).
- 14.
Rossi, M., Mason, D., Chen, J., Tsaturyan, Y. & Schliesser, A. Measurement-based quantum control of mechanical motion. Nature 563, 53–58 (2018).
- 15.
Rossi, M., Mason, D., Chen, J. & Schliesser, A. Observing and verifying the quantum trajectory of a mechanical resonator. Phys. Rev. Lett. 123, 163601 (2019).
- 16.
Belavkin, V. P. Quantum filtering of Markov signals with white quantum noise. In Quantum Communications and Measurement (eds Belavkin, V. P. et al.) 381–391 (1995).
- 17.
Iwasawa, K. et al. Quantum-limited mirror-motion estimation. Phys. Rev. Lett. 111, 163602 (2013).
- 18.
Wieczorek, W. et al. Optimal state estimation for cavity optomechanical systems. Phys. Rev. Lett. 114, 223601 (2015).
- 19.
Setter, A., Toroš, M., Ralph, J. F. & Ulbricht, H. Real-time Kalman filter: cooling of an optically levitated nanoparticle. Phys. Rev. A 97, 033822 (2018).
- 20.
Liao, J. et al. FPGA implementation of a Kalman-based motion estimator for levitated nanoparticles. IEEE Trans. Instrum. Meas. 68, 2374–2386 (2019).
- 21.
Sudhir, V. et al. Appearance and disappearance of quantum correlations in measurement-based feedback control of a mechanical oscillator. Phys. Rev. X 7, 011001 (2017).
- 22.
Tebbenjohanns, F., Frimmer, M., Jain, V., Windey, D. & Novotny, L. Motional sideband asymmetry of a nanoparticle optically levitated in free space. Phys. Rev. Lett. 124, 013603 (2020).
- 23.
Kamba, M., Kiuchi, H., Yotsuya, T. & Aikawa, K. Recoil-limited feedback cooling of single nanoparticles near the ground state in an optical lattice. Phys. Rev. A 103, L051701 (2021).
- 24.
Windey, D. et al. Cavity-based 3D cooling of a levitated nanoparticle via coherent scattering. Phys. Rev. Lett. 122, 123601 (2019).
- 25.
Delić, U. et al. Cavity cooling of a levitated nanosphere by coherent scattering. Phys. Rev. Lett. 122, 123602 (2019).
- 26.
de los Ríos Sommer, A., Meyer, N. & Quidant, R. Strong optomechanical coupling at room temperature by coherent scattering. Nat. Commun. 12, 276 (2021).
- 27.
Delić, U. et al. Cooling of a levitated nanoparticle to the motional quantum ground state. Science 367, 892–895 (2020).
- 28.
Gieseler, J., Novotny, L. & Quidant, R. Thermal nonlinearities in a nanomechanical oscillator. Nat. Phys. 9, 806–810 (2013).
- 29.
Frimmer, M. et al. Controlling the net charge on a nanoparticle optically levitated in vacuum. Phys. Rev. A 95, 061801 (2017).
- 30.
Tebbenjohanns, F., Frimmer, M. & Novotny, L. Optimal position detection of a dipolar scatterer in a focused field. Phys. Rev. A 100, 043821 (2019).
- 31.
Seberson, T. & Robicheaux, F. Distribution of laser shot-noise energy delivered to a levitated nanoparticle. Phys. Rev. A 102, 033505 (2020).
- 32.
Vamivakas, A. N. et al. Phase-sensitive detection of dipole radiation in a fiber-based high numerical aperture optical system. Opt. Lett. 32, 970–972 (2007).
- 33.
Clerk, A. A., Devoret, M. H., Girvin, S. M., Marquardt, F. & Schoelkopf, R. J. Introduction to quantum noise, measurement, and amplification. Rev. Mod. Phys. 82, 1155–1208 (2010).
- 34.
Abbott, B. et al. Observation of a kilogram-scale oscillator near its quantum ground state. New J. Phys. 11, 073032 (2009).
- 35.
Bushev, P. et al. Shot-noise-limited monitoring and phase locking of the motion of a single trapped ion. Phys. Rev. Lett. 110, 133602 (2013).
- 36.
Doherty, A. C. & Jacobs, K. Feedback control of quantum systems using continuous state estimation. Phys. Rev. A 60, 2700–2711 (1999).
- 37.
Poggio, M., Degen, C. L., Mamin, H. J. & Rugar, D. Feedback cooling of a cantilever’s fundamental mode below 5 mK. Phys. Rev. Lett. 99, 017201 (2007).
- 38.
Safavi-Naeini, A. H. et al. Observation of quantum motion of a nanomechanical resonator. Phys. Rev. Lett. 108, 033602 (2012).
- 39.
Braginskiĭ, V. B. & Vorontsov, Y. I. Quantum-mechanical limitations in macroscopic experiments and modern experimental technique. Sov. Phys. Uspekhi 17, 644–650 (1975).
- 40.
Ranjit, G., Cunningham, M., Casey, K. & Geraci, A. A. Zeptonewton force sensing with nanospheres in an optical lattice. Phys. Rev. A 93, 053801 (2016).
- 41.
Monteiro, F. et al. Force and acceleration sensing with optically levitated nanogram masses at microkelvin temperatures. Phys. Rev. A 101, 053835 (2020).
- 42.
Moore, D. C. & Geraci, A. A. Searching for new physics using optically levitated sensors. Quant. Sci. Tech. 6, 014008 (2021).
- 43.
Monteiro, F. et al. Search for composite dark matter with optically levitated sensors. Phys. Rev. Lett. 125, 181102 (2020).
- 44.
Carney, D. et al. Mechanical quantum sensing in the search for dark matter. Quant. Sci. Tech. 6, 024002 (2020).
- 45.
Leggett, A. J. Testing the limits of quantum mechanics: motivation, state of play, prospects. J. Phys. Condens. Matter 14, R415–R451 (2002).
- 46.
Chen, Y. Macroscopic quantum mechanics: theory and experimental concepts of optomechanics. J. Phys. At. Mol. Opt. Phys. 46, 104001 (2013).
- 47.
Genoni, M. G., Zhang, J., Millen, J., Barker, P. F. & Serafini, A. Quantum cooling and squeezing of a levitating nanosphere via time-continuous measurements. New J. Phys. 17, 073019 (2015).
- 48.
Ralph, J. F. et al. Dynamical model selection near the quantum-classical boundary. Phys. Rev. A 98, 010102 (2018).
- 49.
Rakhubovsky, A. A. & Filip, R. Stroboscopic high-order nonlinearity in quantum optomechanics. Preprint at https://arXiv.org/abs/1904.00773v1 (2019).
Acknowledgements
We thank J. M. Leitão for his introduction to optimal control, and P. Vezio, H. Hepach and T. Westphal for discussions and their help in the laboratory. L.M. thanks A. Rauschenbeutel for the discussion inspiring the confocal detection scheme. This project was supported by the European Research Council (grant agreement no. 649008, ERC CoG QLev4G), by the ERA-NET programme QuantERA under grants QuaSeRT (contract no. 11299191) and TheBlinQC (project no. 731473) (via the EC, the Austrian ministries BMDW and BMBWF and research promotion agency FFG), by the European Union’s Horizon 2020 research and innovation programme under grant no. 863132 (iQLev), and by the Austrian Science Fund (FWF, START Project TheLO, Y 952-N36). L.M. is supported by the Vienna Doctoral School of Physics (VDS-P) and by the FWF under project W1210 (CoQuS).
Author information
Affiliations
Contributions
L.M. designed and built the experiment, P.R. designed and programmed the filter and controller. L.M. and C.B. performed the measurements. L.M., P.R. and C.B. analysed the data. The work was supervised by A.K. and M.A. All authors discussed the results and contributed to writing the paper.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature thanks the anonymous reviewers for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
This file contains Supplementary Text, Supplementary Figures 1 – 13, Supplementary Equations 1 – 104 and Supplementary References.
Rights and permissions
About this article
Cite this article
Magrini, L., Rosenzweig, P., Bach, C. et al. Real-time optimal quantum control of mechanical motion at room temperature. Nature 595, 373–377 (2021). https://ift.tt/2UQynzn
-
Received:
-
Accepted:
-
Published:
-
Issue Date:
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.
"control" - Google News
July 14, 2021 at 10:02PM
https://ift.tt/3ibAeqM
Real-time optimal quantum control of mechanical motion at room temperature - Nature.com
"control" - Google News
https://ift.tt/3bY2j0m
https://ift.tt/2KQD83I
Bagikan Berita Ini















0 Response to "Real-time optimal quantum control of mechanical motion at room temperature - Nature.com"
Post a Comment